feature
Don’t Force It!
Using guiding questions to scaffold kindergartner’s thinking of pushes and pulls
Science and Children—November/December 2021 (Volume 59, Issue 2)
By Jesse Wilcox, Naryah Moore, Sarah Nolting, Courtney Reyna, and Caitlyn Potter

Asking questions is a crucial way teachers learn about student’ thinking about science concepts. However, for students to understand and answer teacher questions, they must understand the structure, meaning, and terminology embedded within the question (Lee and Eskritt 1999). While some early childhood teachers may be tempted to ask yes/no questions to reduce the complexity of the questions, Fritzley and Lee (2003) found that young children tend to have biases in how they answer yes/no questions that can obscure what they actually know and can do. Further, Voss, Kruse, and Kent-Schneider (2020) found yes/no questions do not elicit as much information from students as more open-ended questions. How, then, can early childhood teachers ask developmentally appropriate questions? One way to scaffold children’s thinking is to use the HRASE (History, Relationships, Application, Speculation, Explanation) strategy (Wilcox and Kruse 2012; Penick, Crow, and Bonnstetter 1996). This strategy can help probe young children’s thinking and scaffold them toward understanding developmentally appropriate science concepts. In using this strategy alongside a 5E learning cycle (Engage, Explore, Explain, Evaluate, Elaborate), we have found we tend to use speculation, history, and application questions earlier in the 5E relationship and generalized explanations later in the 5E. Therefore, we have rearranged the letters of HRASE to make “SHARE” (Table 1). Importantly, teachers should not be limited to a particular type of question during a particular 5E phase but instead use the open-ended question that will best elicit students’ ideas or scaffold their thinking.
To demonstrate how we scaffold students’ thinking using the SHARE strategy, we embedded questions into a kindergarten modified 5E lesson on pushes and pulls (partially addressing K-PS2-1). This 5E has two Explore and Explain phases because students test two variables. While the 5E is modified, we still start with concrete experiences that serve as a context to ask students questions about pushes and pulls.
Engaging Students with a Ramp and a Cup
To begin this lesson, we start with an anchor phenomenon using pictures and videos of children sledding. We have pictures of the same children on two hills. We ask students, “What do you think will be different when the kids go down the big hill vs. the small hill?” (Speculation). Students often accurately predict the kids will go faster down the big hill. We then show a picture of two children in a sled and another child in another sled on the same hill. We ask, “What do you think will happen when two kids race one kid down the hill?” (Speculation). Students’ predictions are often mixed. We then tell students we are going to model this experience with ramps.
We set up a ramp with a cup near the bottom of the ramp while students are seated on the carpet (Figure 1). We ask students “What do you think will happen when we roll the ball down the ramp into the cup?” (Speculation). Students often say, “The ball will push the cup!” and “The cup will move too!” We roll the ball down the ramp and students are delighted that their predictions were accurate. We then pose a question: “What could we do to get the cup to move farther?” (Relationship). Students discuss with their “elbow partner” for about a minute and then we discuss their ideas as a whole group. Students often say, “We could get a heavier ball,” “We could push the ball,” and “We could make the ramp go higher.” We write their ideas on the whiteboard, and then we pick one of the ideas to test first. We often start with the mass of the ball because in our experience students make the connection between mass and push more readily than speed.
| Table 1. SHARE Strategy (Modified from Penick, Crow, and Bonnstetter 1996). | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
|

Ramp setup.
Exploring the Mass of the Ball (Explore #1)
To test the effect of mass on a ball, we show students three balls that are similar in size but have different mass, such as a Ping-Pong ball, a bouncy ball, and a golf ball. We often pass around the different balls and have students speculate what will happen when they roll them down the ramp. We ask, “How do you think the cup will move if we roll the heavy ball down the ramp?” (Speculation). Students often predict “It will go far!”
Prior to students exploring changing the mass of the ball, we discuss how we could keep track of each trial. We ask students, “What could we do with the butcher block paper to help us remember what ball we rolled and where the cup landed?” (Application). Students come up with answers such as, “We can mark it!” and “We can write on the paper.” We then ask, “Why might we want to use three colors?” (Speculation). Students say they could use a color for each type of ball. We give them a key (Figure 2) where they can color which height corresponds to which color.
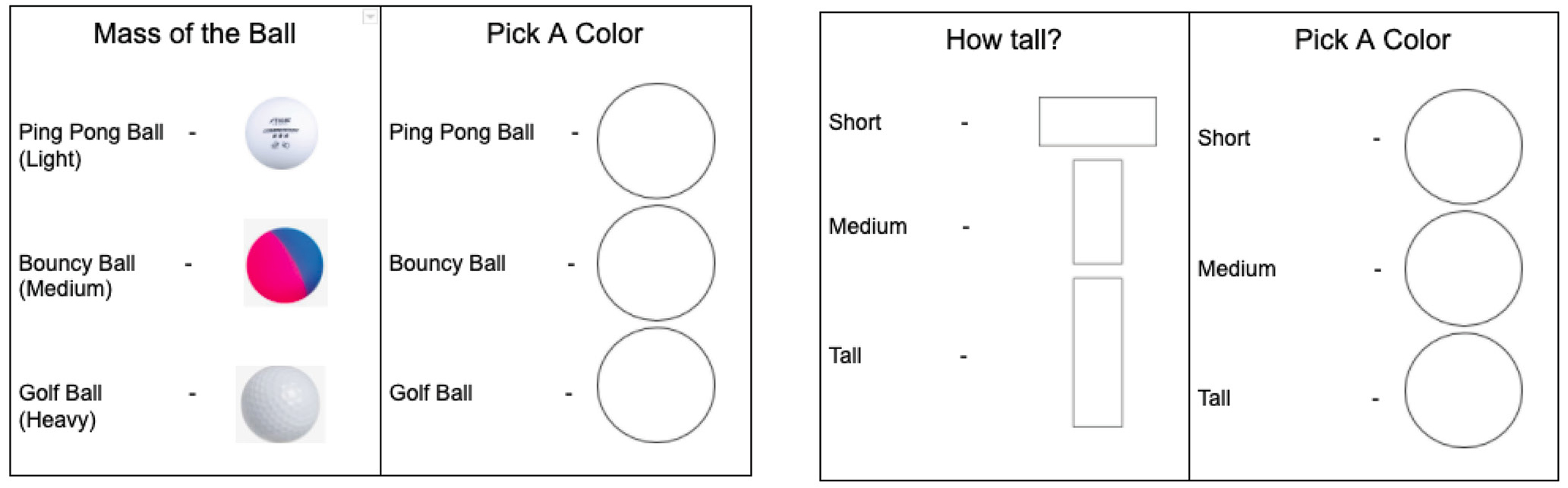
Key for height and mass ramp tests.
Students explore how the mass of the ball affects the distance the cup travels in groups of two or three. Often, we work to encourage collaboration and teamwork by encouraging students to each have a role during the activity, such as rolling the ball, resetting the setup, and marking the cup distance on the paper. As the students explore, we walk around, listen to conversations, and differentiate where needed. We often hear students say things like, “This ball made the cup go really far!” or “This cup didn’t go as far this time.” After their trials, we bring students back to the carpet to discuss their findings.
Explaining the Mass of the Ball (Explain #1)
When students move to the carpet, we display one of the pieces of butcher block paper, which serves as a sort of graph for the students to see. We then help students debrief the activity by pointing to the “graph” and asking, “What do you notice happened to the cup when we used different balls?” (History). Students often quickly note the golf ball pushed the cup the farthest, followed by the bouncy ball and then the Ping-Pong ball moving the cup the shortest distance.
To help guide their thinking, we ask, “What do you notice is different about each ball?” (Relationship), prompting the students to note the differing masses. We ask, “How could we find out which ball has more mass?” Students often suggest holding two of them at once and comparing them. We then bring out a balance and ask them “What do you remember about the balance from math?” (History). Students note they used it to compare the mass of blocks. We then ask, “How could we use this balance from math to help us compare the balls?” (Relationship). Students quickly note they could put a ball on each side and figure out which one has the most mass.
After students determine the relative mass of the balls, we ask, “How does the mass of the ball change how the cup moves?” (Explanation) and “Students respond with, “The heavy ball made the cup go far, but the light ball only made the cup move a tiny bit.” We continue to discuss the students’ findings. Once students come to an agreement, we help them summarize, “The heavier the ball, the farther the cup is pushed.”
Exploring the Ramp Height (Explore #2)
After students have an understanding of how the mass of the ball affects the push, we explore how different ramp heights affect speed. We use precut wooden ramp heights of 6 cm, 9 cm, and 12 cm. To begin the activity, we show students three different pieces of wood used to adjust the ramp height (we call them short, medium, and long with the students). Holding up the different sizes of wood, we ask, “What do you think will happen to the cup if we change the height?” (Speculation). Students say “I think it will go pretty far with the tall one!” and “The short one won’t move it as far.” We explain we will use the same method of tracking the tests as we did when we tested the effect of the ball’s mass by using butcher block paper and marking the distance the cup traveled with different colors for the different heights (short, medium, tall). We again provide a key (Figure 2) where students can color which height corresponds to which color.
Students explore how the height affects the distance the cup travels in groups of two or three. We continue to encourage collaboration and teamwork by encouraging students to each have a role during the activity (changing height, marking cup, rolling the ball). While students are working, we again move around the room and differentiate by scaffolding students’ thinking through questions and assisting students who need help changing the ramp height.

Explaining the Ramp Height (Explain #2)
Once students have completed their tests, we bring them back to the carpet to discuss as a whole group for about 10 minutes. We start by looking back at what they thought would happen, remembering they predicted the highest ramp would go the farthest. We then ask the students, “What happened to the cup when you changed the height of the ramp?” (History). Students responded that the highest ramp did make the cup go farthest and that the higher the ramp, the farther the cup was pushed.
We continue to guide the students’ thinking further by showing students all three ramp heights at once. We roll a ball down each of them at the same time and allow students to notice what happens. We ask, “What happened when we rolled the balls at the same time?” (History). Students typically respond, “The ball on the tall ramp went faster.” We then ask, “If the ball is going faster, what does it do to the cup?” (Relationship). Students reply, “It hits the cup harder.”
Guiding questions like these allowed students to think critically about why the outcomes are occurring, rather than only observing. We continued to make connections between their findings to deepen their understanding. Finally, we guide students to develop a summary of the activity. They typically say, “A higher ramp makes the ball go faster” and “A fast ball pushes the cup more.”
Finally, we return to the sled anchoring phenomenon we started with in the Engage phase. We show students their initial predictions and ask if they still agree. Most students still agree that the larger hill will make the kids go faster and often mention the same thing happened with the ramps. We then show the picture with two children in one sled and another child in another sled. Students accurately explain the sled with two children should go faster down the hill because they would be heavier.
Elaborating Using a Pull Activity
As an elaboration, we show an empty basket with a string attached and we demonstrate pulling the basket (Figure 3). After students observe and process the information, we ask, “What do you think would happen if we added dominoes into the basket and then pulled it?”(Speculation). The students responded, “It would get heavy.” At this point, we have students test this in groups of two.

Pull activity with basket.
We have students explore the materials for a few minutes focusing on how adding or subtracting dominoes affects pulling the basket. While students explore, we ask questions such as: “What did you feel when you changed the number of dominoes?” (History). We then direct their attention to the graphic organizer (Figure 4). We explain that they will pull the basket with a certain number of dominoes inside, then after they pull the basket, they will mark on the spectrum line how easy or hard it was to pull the basket. If it was really easy, they will mark it on the far left side, and if it was really hard, they would mark it on the far right side. Students then start the investigation.
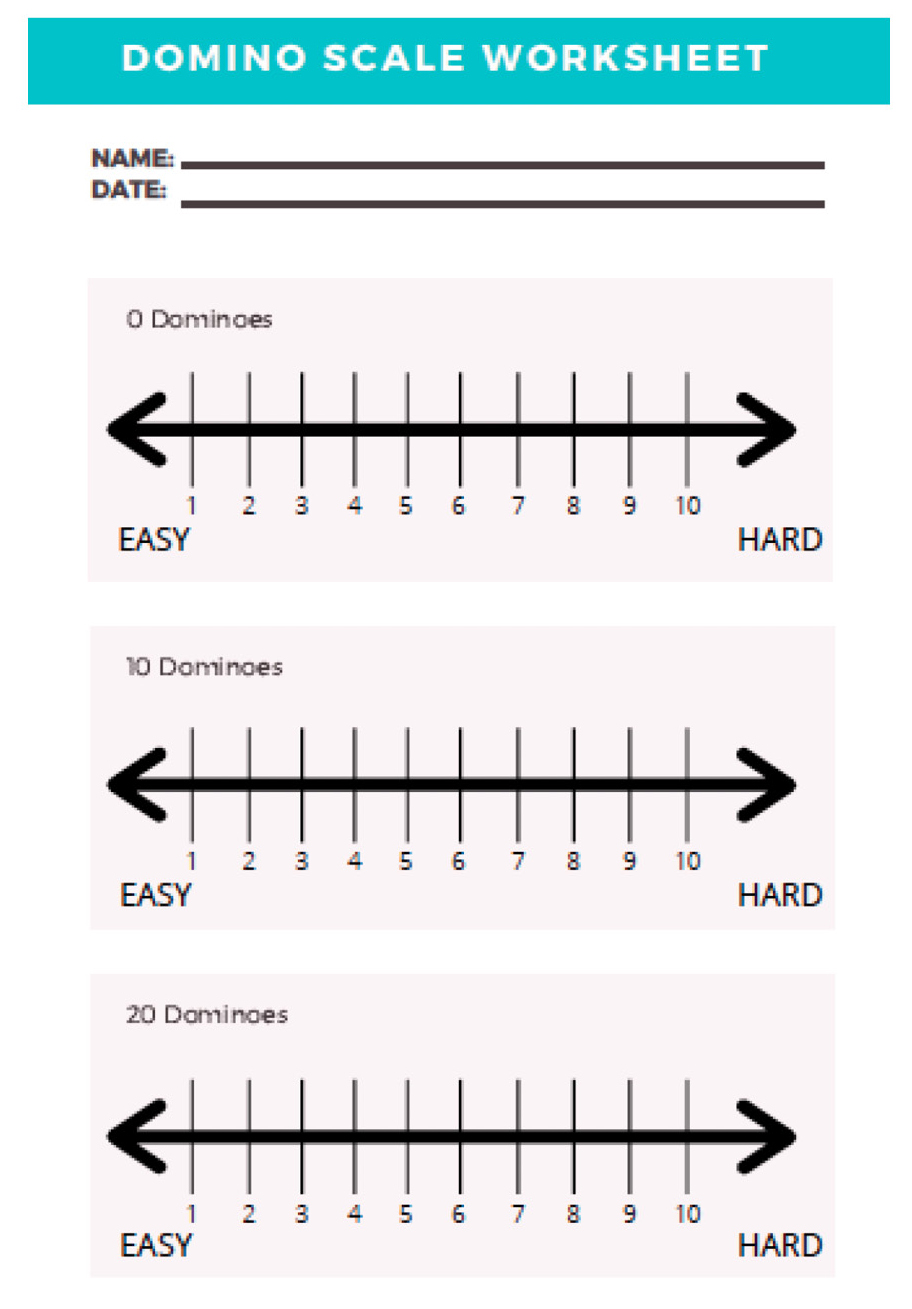
Domino graphic organizer.
After the students finish their graphic organizers, we go through each line for the students to share what they marked and why. On the whiteboard, we make a line and mark all the students’ results, using a different color for the different tests. With the class, we discuss what the scale showed us. We ask, “What do you notice happens as we add more dominoes?” (Explanation). Students note that the heavier the basket is, the harder they had to pull.
Evaluating Pushes and Pulls
To assess students’ understanding of pushes and pulls, we give pairs of students 12 pictures of everyday activities such as pushing a grocery cart, zipping a coat, and a parent pushing a child on the swing. We have children sort these activities into two groups, but we don’t tell the children the names of the groups. As children are sorting, we walk around and listen carefully to their discussions and ask questions. Even though we are assessing students and documenting their answers on a checklist, we still engage them with SHARE questions to scaffold their thinking when necessary. Many students sort the tasks into pushes and pulls, but some groups use other categories such as indoor/outdoor. We ask scaffolding questions such as:
- What are some ways you could sort these objects? (Speculation)
- What do you remember learning about pushes and pulls? (History)
- How could you use what you learned before to help you sort these? (Application)
- How is this picture like what we did with the ramps? (Relationships)
- Why does the way you organized these make sense? (Explanation)
After students are finished sorting, we do a gallery walk where one student from the group stays and one “strays.” Students talk with each other about how they organized their groups and return to their original partners after a few rounds of sharing. We ask pairs of students to evaluate how other groups organized their pictures. After about a minute of discussion, we have students come together in a large group. We ask, “What are the different ways you saw groups organize their pictures?” (History). Students quickly recall the organizational strategies. We then ask, “If we wanted to organize the pictures based on what you learned in class, what could you do?” (Relationship). Students quickly note they could organize the pictures by pushes and pulls. We then ask, “How can you tell if the picture is a push or a pull?” (Explanation). Students often talk about the way the object moves (e.g., if it moves toward you or away from you). Students then make adjustments to their categories. For students that are already finished, we differentiate by asking them to organize the pictures in each category from smallest to biggest push/pull (similar to Figure 4).
Conclusion
In this kindergarten lesson about pushes and pulls, we used the SHARE strategy to embed effective questions throughout each phase of the 5E. These thoughtfully worded and open-ended questions can help students generate ideas and focus their thinking (Clough 2007). Additionally, these questions can help teachers understand students’ thinking and scaffold accordingly. Effective questions are an essential part of effective teaching children of any age. ●
Acknowledgments
We’d like to thank Elyse Webb for hosting us in her kindergarten class. We would also like to thank the Iowa Science Foundation for funding this project.
Jesse Wilcox (jesse.wilcox@uni.edu) is an assistant professor in biology and science education at the University of Northern Iowa in Cedar Falls, Iowa. Naryah Moore, Sarah Nolting, Courtney Reyna, and Caitlyn Potter are all undergraduate elementary education students at Simpson College in Indianola, Iowa.
Phenomena Physical Science Physics Grade K

